Configurations of planet

Harmony of spheres

Pythagoras considered his discovery - that sounds whose frequencies are in the ratio of small integers create consonant (euphonious) consonance - to be so fundamental that dared him to generalize to movements in the whole universe. According to Pythagoras, the planets creates harmony - "harmony of the spheres". But we do not hear the sound of this cosmic harmony, because we had been accustomed to it since our birth ...

The first who managed to move the Pythagorean idea a bit further was Johannes Kepler. J.Kepler examined the ratios of distances of the planets from the Sun (he tried to inscrib and circumscribe some regular bodies to spheres of planetary motion) and the ratios of velocities of the planets. He believed that each planetary sphere belongs to one tone of scale.

J.Kepler finally found certain ratios close to integer fractions - ratios of velocities of planets in the perihelion and aphelion. However speeds of planets in the perihelion and aphelion depends on the eccentricity of elliptical orbit of the planet and it changes over time. E.g. Planet Mercury has today eccentricity c. 0.206, but its value may vary about in the limits from 0.121 to 0.232 (and similarly fluctuates also inclination of orbit). It is unlikely that there could be integer ratios between variables that change over time (in the absence of direct dependence of these changes, ...).

Titius’ rule

J.D.Titius replaced (y.1766) Kepler's complicated polyhedra by a simple rule - he expressed the size of the large half-axis (a) as an exponential function of the ordinal number of planet (k):

 a ≈ 0.4+0.3*2^k [AU] 

For k=0,1,2,4,5 Titius formula gives approximate distance for Venus, Earth, Mars, Jupiter and Saturn from the Sun.

After publishing by J.E.Bode (y.1772) in popular astronomy book the rule became known as Bode's Law. After the discovery of Uranus (y.1781) on the orbit corresponding to k=6 the attention of astronomers centered to the orbit k = 3 in the band between Mars and Jupiter, where was (y.1801) discovered asteroid Ceres and later several other smaller bodies - asteroids.

The original Titius' proposal foresaw also a distance of 0.4 AU for Mercury, In this case, however, (as was shown by Charlier) the said series is not geometric, respectively it gives for Mercury k=-∞.

For k=-1,-2,… between Mercury and Venus no body was found and thus for Mercury Titius series fails.

S.F.Dermott (y.1973) showed, that a set of randomly generated periods can be under certain conditions covered by the simmilar rule as the Titius one and questioned so that the planetary orbits are on a logarithmic scale nonrandom.

Generalizations

Mary A.Blagg (1913) attempted by calculations based on the relationship

 a ≈ c₁+ c₂ z^k [AU] 

cover not only the system of the planets but also satellite systems of Jupiter, Saturn and Uranus.

In pursuit of the best possible coverage of known data for value c₁ she set a complicated formula (containing goniometric functions,…)

A.Mohorovičič (1938) modified the Titius rule to form:

 a ≈3.363± 3.363*0.88638^k [AU] 

with which he tried to describe also some well-known asteroids and comets. Mohorovičić identified some basic conditions which the right relation would have, e.g. it should cover the Sun and stable tracks of asteroids and comets (elements with variable track need not be covered by the relation, ...).

According to Mohorovičič's rule the greatest incidence of bodies is in distance c. 3.363 AU, i.e. in band of asteroids and number of spheres from this point decreases to both sides. Mohorovičič's proposal is quite acceptable for inner system of planets (to which asteroids Eros and Hermes are incorporated before Mars) and for asteroids between Mars and Jupiter. However, for the outer planets spheres with these numbers are formed: 5 (Jupiter) -5 (Saturn) -13 (Uranus) and -17 (Neptune), ...

The result of M.A.Blagg and Mohorovič's efforts is a relatively extensive set of orbits, but it does not cover the whole diversity of the Solar systems.

Attempts to exact solution

According V.Guth and F.Linka (y.1947) the regularity of distances of the planets and many months can not be accidental. Distances result from mutual gravitational pull of the central body and the bodies orbiting around it. Internal interplay of gravitational forces acts that -after a certain time- the distance of bodies stabilize to the values describable by some mathematical relationship.

M.W.Ovenden (y.1972) suggested explanation for the Titius rule (Bode law) dynamically - neighboring planets can not be too close. How far should planets be at least may depend on their weights (which would also explain the observed differences of distances between smaller inner planets and larger outer planets).

Arrangement of bodies

The bodies in systems are either equivalent, e.g binaries (such as covalent binding in chemistry, ...) or some of the bodies is stronger (heavier) and determines the behavior of other bodies (ionic binding, ...).

Solar system is system with a strong central body (the Sun) and several slave centers.

Types of arangement

According to the position of the smaller body relative to the larger (and central) body we distinguish two types of arrangements:

                                Central - Smaller - Bigger
      +----+
      |    |       +---+
      |    |  +-+  |   |
      +----+  +-+  +---+
·   the Sun - Mercury - Venus - Earth - Jupiter
·   Jupiter - Io - Europa - Ganymed
·   Saturn - Mimas - Enceladus - Tethys - Dione - Rhea - Titan
·    Uran - Miranda - Ariel - Titan
·    Earth – artificial satellites -Moon 

                            Central - Bigger - Smaller
      +----+
      |    |  +---+
      |    |  |   |   +-+
      +----+  +---+   +-+
·   the Sun - Jupiter- Saturn - Neptune - Pluto
·   Jupiter - Ganymed- Kallisto
·   Saturn - Titan - Japetus
·   Uranus - Titania - Oberon
·   Mars - Phobos - Deimos 

Exceptions to these schemes are:

• bodies on the same track, e.g. Sun-Trojans/Jupiter, Saturn-Helene/Dione
• bodies about the same size, e.g. Sun-Venus/Earth

Bodies according to influence

Depending on the distance from its center and the distance from the center of the superior system the following cases are distinguished:

• Bodies close to the center (Mars: Phobos, Deimos, Jupiter: the nearest moons)
• Bodies interfered with by other bodies (Laplace's resonance,…)
• Bodies systems disturbed by the center (distant moons of the Jupiter,…)

Symmetry of motion

Motion of geometrical axes

Geometrical axis

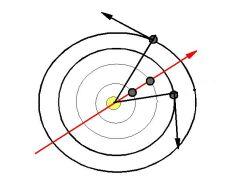
Let us consider motion of two bodies P,Q around center S and motion of thought (undirected, geometrical) axis A of angle PSQ. At time of planetary conjunction P-Q, axis A is aligned with line SP =SQ. During time t the first line cover (with regard to the original line) angle t/P (*360°), meanwhile the second line angle t/Q (*360°).
The axis A cover (during time t) angle:
t/Q+(t/P-t/Q)/2 = t/Q+t/(2P)-t/(2Q) = t *(1/(2P)+1/(2Q)) (*360°).

Axial period is period, after which the axis of angle P-S-Q align with its original position. Axial period of two orbital periods P, Q is period:

 [P,Q] = 2/(1/P+1/Q)= 2∙P∙Q/(Q+P) 

We designate axial period with brackets [].

During time [P,Q] axis runs through full angle 360°.

For any periods A,B,C and constant k it holds:

Considering pair of planets P,Q and the Sun, center of mass is always somewhere inside the triangle SunPQ. If we neglect differences in masses and in actions, we can assume, center of mass move in step with axis of angle PsunQ, i.e. with period [P,Q].

We can imagine axial period (in contrast to synodic period) as an orbital period; (and we can, as the case may be, compare it with other orbital periods).
Gravity centre of (three) bodies moves (around S) approximately with axial period.

Axial period of more bodies

Axial period of n bodies having periods P,Q,R,S... is determined by harmonic average of given periods:

 [P,Q,R,S,...] = n/(1/P+1/Q+1/R+1/S...) 

    [J,S]= 16.91324 years ( 6177.56 days)
    [J,U]= 20.78898 years ( 7593.18 days)
    [J,N]= 22.13075 years ( 8083.26 days)
    [S,U]= 43.62101 years (15932.57 days)
    [S,N]= 49.97918 years (18254.89 days)
    [U,N]=111.29089 years (40649.00 days)

    [M,V]=0.346170 years (126.4385 days)
    [M,E]=0.388199 years (141.7896 days)
    [M,R]=0.427013 years (155.9666 days)
    [V,E]=0.761766 years (278.2351 days)
    [V,R]=0.927141 years (338.6381 days)
    [E,R]=1.305775 years (476.9343 days)

Symmetrical configurations

Configuration of more bodies is axially symmetric, when certain axes are identified into one axis. In case of even number of bodies such situation occurs, when all axes of selected pairs of bodies align. Alignment of two axes can be at angle 0° or 180°, so period of alignment is half of synodic period computed from periods of these axes. 

    Date    (Interval) Math.date
    -------------------------------------
    2014 Jun 10 AD  ( 9.69)  2014.45     JS-UN   
    2024 Aug 30 AD  (10.22)  2024.67   
    2034 Jul 20 AD  ( 9.89)  2034.56
    2044 Aug 31 AD  (10.12)  2044.67
    -------------------------------------
    2018 May  6 AD  (18.56)  2018.35     JU-SN
    2036 Feb 23 AD  (17.80)  2036.15
    ------------------------------------- 
    2023 May 29 AD  (22.08)  2023.41     JN-SU
    2046 Jul 13 AD  (23.12)  2046.54

Mayan period tzolkin (z) in symmetric configurations (as quantum):

Symmetric configurations	Mayan period (multiple of tzolkin)	Note
([J,S],[U,N]) = 19.944 years= 7284.6 days	7280 days = 28*z= 20*364 days = 260*28 days (260 natural months) 7280 days = 21*346.67 days (c. 21 ecliptic years)	(J,S)= 7253.5 days = 19.86 years
([J,U],[S,N]) = 35.594 years = 13001 days	13000 days =50*z= 35.59 years 12997.8 days =3*J = 35.59 years	(S,N)= 13101.5 days = 35.87 years.

Motion of axes of inner planets measured with regard to axes [J,S] results in periods (n² multiples of quantum q=16.2 days):

([M,V], [J,S]) = ( 63.219, 3089.0) = 64.540 d (2²*q) ([V,E], [J,S]) = (139.118, 3089.0) =145.679 d (3²*q) ([E,R], [J,S]) = (238.470, 3089.0) = 258.420 d (4²*q) ~ tzolkin.

Period (V,E)= 583.92 d (6²*q).

Inverse motion

Periodical symmetry

Let us have line r moving with period R around centre. With regard to line r bodies P and Q move inversely if r is oriented axis of motion of these two bodies, i.e. when R=[P,Q].
Bodies open and then close angle with regard to r with synodic period (P,Q).

Let us assume, it is not necessary that this motion run continuously. Let the condition of inverse holds at every instant t = k*T, where k is whole number and T time interval (period). At every instant t it holds:

 Lp - Lr = Lr - Lq 

where Lp,Lq and Lr are longitudes of bodies P,Q and Lr is longitude of line r. So
frac(T/P)-frac(T/R) = frac(T/R)-frac(T/Q), i.e. 2*frac(T/R) = frac(T/P)+frac(T/Q)

Barycentric periods

Two bodies (binary stars) orbit around a common center of gravity with the same periods.
In the case of more bodies the situation complicates.

Center of gravity is -during motion of two planets around the Sun (neglecting the difference in mass of the planets)- always on the geometrical axis of links of planets with the centre - see axial period. But it (the center of gravity) is moving with axial period only close to the conjunctions of planets.

During oppositions - "singularities" occurs. At the moment of singularity -physically- nothing special happens - orientation of axis overshoots. So motion of centroid axes recalls rather the wave behavior of atoms, than a simple cyclical motion with a fixed period.

Irregular motion of real axes

Angular velocity of motion of the center of gravity varies, movement of real oriented centroid axes is irregular.

At the moment of opposition the "polarity reversal", i.e. a sudden change in direction of the axis, appears. Centroid axis movement slows and then changes the direction from the central body to the center of gravity by 180º. These "singularities" make it difficult to calculate mean periods of oriented axes motion.

Computation of "instant periods" in conjunctions

dx = dx₁m₁+dx₂m₂ / (m₁+m₂), where dx_i = a_i cos dϕ_i, dy_i = a_i sin dϕ_i, dϕ_i = dt/P_i

E.g. for Jupiter (J) and Saturn (S), dt=1 year (after conjunction J-S), a_J=5.202 AU, aS = 9.535 AU.
dϕJ = dt/P_J = 30.35°
dx_J = 5.202 * cos(30.35°) = 4.489 AU   dy_J = 5.202 * sin(30.35°) = 2.628 AU
dϕ_S = dt/P_S = 12.22°
dx_S = 9.535 * cos(12.22°) = 9.319 AU   dy_S = 9.535 * sin(12.22°) = 2.018 AU

Σ mi dxi = 317.83*dx_J + 95.16*dx_S = 317.83*4.489 + 95.16*9.319 =  2313.5 [Mearth*AU]
dxi = 5.60 AU
Σ mi dy_i = 317.83*dy_J + 95.16*dy_S = 317.83*2.628 + 95.16*2.018 =  1027.3 [Mearth*AU]
dy_i = 2.49 AU

tg(dϕ) = dy_i / dx_i = 2.49 / 5.60 = 0.4446
dϕ = 23.94°
PJS = 1 rok * 360°/23.94° = 15.03 years

    [J,S]= 16.91 years
    [J,U]= 20.79 years
    [J,N]= 22.13 years [J,S,U,N]= 29.36 years
    [S,U]= 43.62 years
    [S,N]= 49.98 years
    [U,N]=111.29 years

    [J,S]= 15.03 years
    [J,U]= 13.49 years
    [J,N]= 15.16 years [J,S,U,N]= 19.03 years
    [S,U]= 34.73 years
    [S,N]= 41.94 years
    [U,N]=123.27 years

Mean period of centroid axis computed at time when bodies are on one half-line (i.e. in conjunction) differ from mean periods computed in other starting positions. From elementary angular shifts we obtain e.g. period c. 15 years in conjunction J-S and c. 14 years in quartile J-S.

The real axial period is determined by the heavier of bodies. At the moment of the opposition of bodies centroid axis is directed to the heavier body. Even in the time of conjunction the centroid axis passes the body. Thus, the real centroid axis copies - although by an uneven movement - motion of the heavier of the two planets.

Minimum interaction

Bodies moving in systems maintained by gravitational attraction, paradoxically manifest a kind of "repulsion". Systems prevents accumulation of mass in one place. During extended interaction system passes through a certain evolution. In the end bodies get synchronized and take a certain optimum and stable configuration. This configuration can - in the case of intersecting orbits - exclude collision of bodies (e.g. the motion of the planets Neptune and Pluto).

Repulsion of bodies

Gravitational attraction of bodies has one quite bit paradoxical consequence. Bodies on orbits (around central body) repulse each other. This phenomenon results from principle of conservation of angular momentum (Alfven).
Electrons on orbits also repulse each other. They have the same charge...

Principle of minimum interaction

After long interaction, bodies form an optimal configuration and move such a way, that certain summary characteristic has extreme.
(According to A.M.Molchanov such synchronization would appear even from action of very week forces.)

For some subsystems of solar system (Io-Europa-Ganymed, satellites of Uranus) Ovenden principle (i.e. variational principle of celestial mechanics) was proved. [Ovenden,1974]. Ovenden principle fails for outer planets.

Ovenden,1974: Ovenden, M.W., Feagin, T., and Graf, O., "On the principle of Least Interaction Action and the Laplacean Satellites of Jupiter and Uranus," Celestial Mechanics Journal, 8(4), 455-471 (1974).

The idea of the principle of minimal interaction:

1/ arbitrarily scattered bodies (understood bodies on random orbits) are synchronized (they regulate phase shifts on tracks mutually), so to have (in long-term scale) the sum of their interactions - which are a function of their real (or angular) distance and weight - minimal.

2/ originally random orbits are modified (while keeping the total energy of system ..), in order to further minimize the total interaction (and new orbits again readjust their phase shifts by 1/)

By 1/ so Galilean moons act

By 2/ e.g. position of Venus and Earth between the Sun and Jupiter had to be gradually adjusted so that some characteristics become balanced?!

Planets in distance

Regarding minimal interaction - configuration of planets in the period 18.8.2003-31.8.2003 is interesting.

Let us mark V-Venus, E-Earth, R-Mars, J-Jupiter, U-Uran and observe heliocentric conjunctions and oppositions.

It seams like distances: J-U, J-E, J-R, E-V, R-V, (U-V), R-Moon maximized in this configuration.

In the moment of nearest approach of Mars to Earth, the adjacent (concurrent) bodies Jupiter and Venus are found on the opposite site of the Solar system.

In the following text we proceed from oppositions J-U, in the year 2003 the exact opposition J-U come till 31.10.2003.

Similar configurations

We find some other similar configurations in years 719.29, 1147.48, 1575.66, i.e. with approximately Babylonian period with regard to 2003.84: 31*(J,U) = 31*13.8123 years =428.18 years.

    12.4.719

    16.6.1147

    17.08.1575

Period 12J

719 - 862 - 1004,

1147 - 1290 - 1433,

1575 - 1718 - 1861,